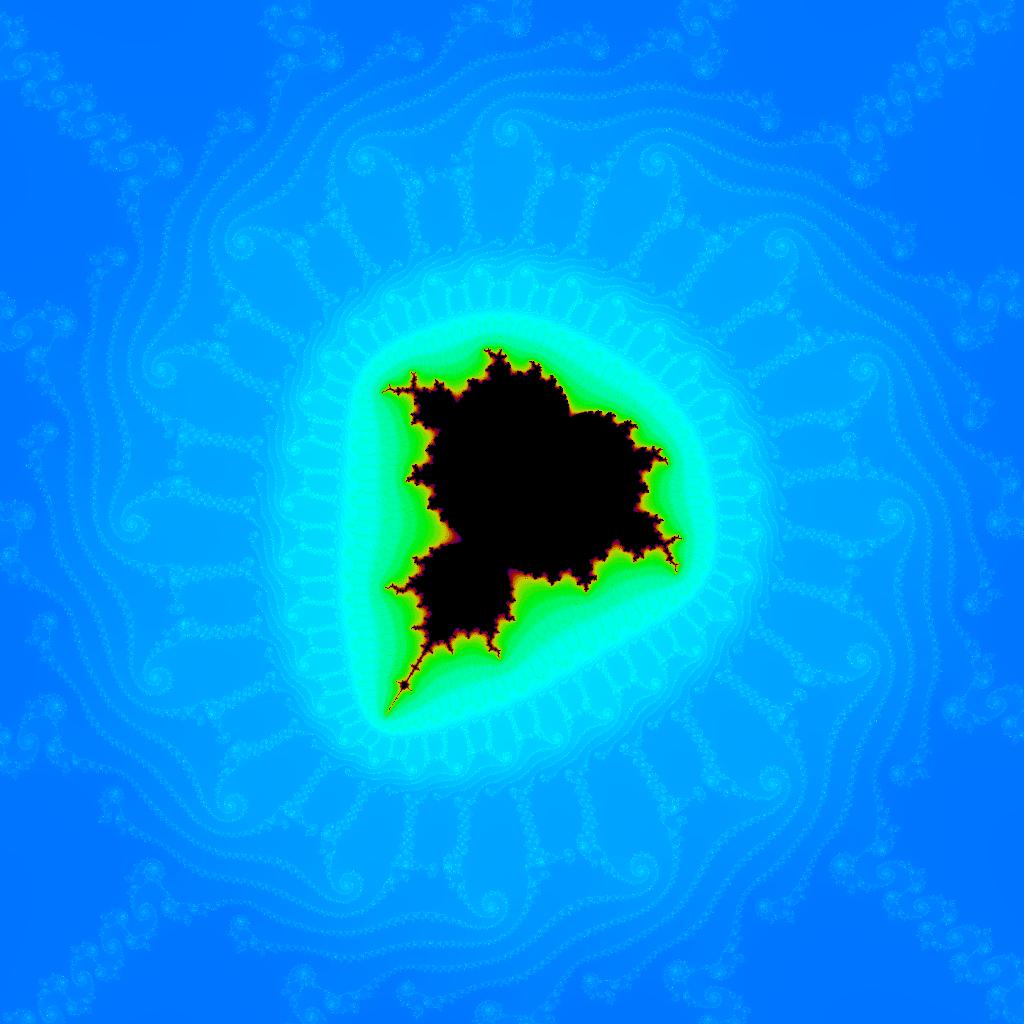
Write a Python program to generate 1000+ images of Mandelbrot Set with ever zooming ranges. Please note that Turtle is not used in this project for speed consideration. Then use an external software to combine the images into a video:
Source Code:
from PIL import Image
import colorsys
import math
px, py = -0.7746806106269039, -0.1374168856037867 #Tante Renate
R = 3
max_iteration = 2500
w, h = 1024,1024
mfactor = 0.5
def Mandelbrot(x,y,max_iteration,minx,maxx,miny,maxy):
zx = 0
zy = 0
RX1, RX2, RY1, RY2 = px-R/2, px+R/2,py-R/2,py+R/2
cx = (x-minx)/(maxx-minx)*(RX2-RX1)+RX1
cy = (y-miny)/(maxy-miny)*(RY2-RY1)+RY1
i=0
while zx**2 + zy**2 <= 4 and i < max_iteration:
temp = zx**2 - zy**2
zy = 2*zx*zy + cy
zx = temp + cx
i += 1
return i
def gen_Mandelbrot_image(sequence):
bitmap = Image.new("RGB", (w, h), "white")
pix = bitmap.load()
for x in range(w):
for y in range(h):
c=Mandelbrot(x,y,max_iteration,0,w-1,0,h-1)
v = c**mfactor/max_iteration**mfactor
hv = 0.67-v
if hv<0: hv+=1
r,g,b = colorsys.hsv_to_rgb(hv,1,1-(v-0.1)**2/0.9**2)
r = min(255,round(r*255))
g = min(255,round(g*255))
b = min(255,round(b*255))
pix[x,y] = int(r) + (int(g) << 8) + (int(b) << 16)
bitmap.save("Mandelbrot_"+str(sequence)+".jpg")
R=3
f = 0.975
RZF = 1/1000000000000
k=1
while R>RZF:
if k>100: break
mfactor = 0.5 + (1/1000000000000)**0.1/R**0.1
print(k,mfactor)
gen_Mandelbrot_image(k)
R *= f
k+=1This program generates over 1000 images and may be quite slow. You can make it faster by dividing the work to multiple programs each doing a portion of those images.